Life at the End of Quantum Tunnels

Recently a biochemistry student told me that her classmates looked like they had seen a ghost when their professor seemingly took a left turn from a lecture on cellular respiration and started to discuss quantum tunnelling. But this 90-year discovery keeps surfacing in different contexts, reminding us that without the tunnelling effect, there would be no life in the universe.

Part of the lecture focused on iron–sulfur clusters, which play a role in the oxidation-reduction reactions of mitochondrial electron transport. The clusters are part of four protein complexes that sequentially shuttle electrons. The latter are ultimately gained from the breakdown of food molecules and are destined for oxygen. In so doing, protons are consumed inside the mitochondrial membrane while others are pumped out, creating a potential difference that helps motor the synthesis of adenosine triphosphate (ATP). Then ATP goes on to facilitate a host of energy-requiring reactions that keep an organism alive.

Each green arrow represents an electron jump due to quantum tunnelling.   http://www.pnas.org/content/107/45/19157/F2.large.jpg

But each time an iron cluster transfers an electron, it does so against a potential energy barrier. How does it do it? Because of the wave-like properties of a tiny particle like the electron, when it’s up against a thin-enough barrier, such as the 2.2 to 3.0 angstrom gaps (0.22 to 0.30 nanometers) shown in the diagram, there is a small but non-zero probability that the electron will be in the gap, and more importantly, also beyond it.  The best way to convince yourself that quantum tunnelling is physically possible is to go through the math and physics, and if you’re interested, it’s found here.  The author does not show every tedious algebraic step, but if you get stuck, I will gladly help in the comments section. It’s great fun while the laundry is being done.

Life involves a struggle against entropy made possible by a continuous energy source. For the planets and presumably moons that harbour life, the most important energy source is fusion from the sun. If you are like me in that you once assumed that the prodigious gravitational force at the core of a sun could provide hydrogen atoms with sufficient energy to overcome Coulombic repulsion and bring about fusion,

Image credit: E. Siegel

then you were also incorrect. It turns out that the kinetic energies are too small by a factor of 1000. So how does fusion take place? Like electrons in iron clusters, hydrogen atoms, although more massive, are small enough, and thanks to gravity, close enough to overcome the thousandfold barrier working against them. So quantum tunnelling is ultimately working with gravity to make stars shine.

The fact that tunnelling probability decreases steeply with lower thermal velocities extends the duration of smaller stars, those weighing less than 1.5 solar masses. This is important in that it gives life enough time to evolve in solar systems with appropriate conditions. One of the prerequisites of life, we imagine, is the presence of water on the surface of a moon or planet. Whether water is out-gassed or brought in via a comet or asteroid, it has to be first synthesized in molecular clouds according to this reaction between molecular hydrogen and hydroxyl radicals:

OH + H₂  →  H + H₂O

The extremely cold temperatures combined with adsorption on dust particles create boundaries small enough for quantum tunnelling to allow the production of molecular hydrogen from its atomic counterparts. There is even evidence that the hydroxyl reaction itself benefits from the same phenomenon.

From deep space back to our bodies, can tunnelling cause unwelcome changes in the DNA molecule? In the double helix or “twisted ladder” of DNA, each nucleotide of one strand of the ladder is attracted to its complement on the other strand by means of a hydrogen bond. A hydrogen bond consists of a lone pair of electrons from one nucleotide attracted to the hydrogen bonded to an oxygen or nitrogen atom of the nucleotide on the other side of the strand.

from Modelling Proton Tunnelling in the Adenine–Thymine Base Pair
A. D. Godbeer , J. S. Al-Khalili * and P. D. Stevenson

But there is a small possibility that the proton (hydrogen without electrons) can overcome the potential energy barrier and end up bonded to the hydrogen-less atom on the other strand. If the effect would be common enough, it could lead to a mutation. It should be noted that this a very active area of research and these authors have concluded that, at least in the adenine-thymine base pair, tunnelling does not occur. Less controversial is the ideas that quantum tunnelling plays a key role in the repair of DNA from ultraviolet damage, specifically in the electron-transfer needed to undo the dimerization of pyrimidines.

If those shocked biochemistry students read this blog, I am not sure that it would erase the “seen-a-ghost” expression from their faces. As educators we don’t often empathize enough with their survival-mode of trying to focus on the “essentials” that will get them through a given course. Quantum tunnelling and quantum phenomena are central ideas, but grasping them rests on an above average foundation of mathematics, physics and chemistry concepts. Is it realistic to assume that most biochemistry freshmen have already acquired that? We have to be patient, fuel them with enthusiasm and make sure that we don’t muddy the waters of key concepts with too much content in our courses.

Other Sources:

• Quantum Tunnelling to the Origin and Evolution of Life – NCBI – NIH
• Barrier Penetration  http://hyperphysics.phy-astr.gsu.edu/hbase/quantum/barr.html (the applet allows you to see quantum tunnelling’s sensitivity to both the particle’s mass and barrier-distance.)
• Quantum Tunnelling in DNA  http://einstein.drexel.edu/~bob/Term_Reports/Megan_Wolfe.pdf

What Do X-Rays Bring to Mind?

What comes to mind when you hear the word x-rays? Is it medical imaging tools, ranging from a simple radiography of a broken finger to an abdominal CT scan? (Incidentally the latter offers an effective radiation dose that’s 31 000 times larger than the former, equivalent to a 10-year dose of what’s naturally accumulated by the human body.) Do you think of how x-rays form: juiced up with high voltage, electrons, accelerated in a vacuum tube, subsequently collide with a metal plate’s atoms, causing, in each affected atom, an inner electron to be ejected and an outer electron to fall in to occupy the vacated level?  Does it make you smile about the impossibility of Superman’s x-ray vision? Do you think of x-ray crystallography, the technique that was used to help elucidate the structure of biological molecules such as insulin, vitamin B-12 and DNA?  Or is it a reminder of how x-rays helped validate the existence of photons?

Sensually and intellectually, light, to paraphrase William Blake, is eternal delight. The highly variable shadows and colors that its visible forms create upon their interaction with matter help evoke a range of emotions. But more objectively what is light? We can define it in a way that emphasizes the electromagnetic spectrum, in the sense that different forms of light energy have different frequencies, which in a vacuum, all move at the unsurpassable speed of 3.0 × 10⁸ m/s . But like all forms of light, x-rays, whose range of frequencies is only surpassed by those of most gamma rays, don’t always behave like waves. Depending on the nature of the experiment, some results can only be predicted if we assume that light consists of photons.

A photon isn’t really a particle because it’s massless, but it’s not a wave either because a photon has momentum. Without that momentum to dislodge electrons, photosynthesis, the photoelectric effect and digital cameras would not exist. Yet in classical physics, momentum is a product of mass and velocity, so how is it possible for something without mass to have momentum?

When a particle’s velocity gets close to that of light, its momentum cannot be accurately represented by the product of mass and velocity. The momentum gets larger than expected by the Lorentz factor. Originally referred to as β in scientific papers of the past century and now symbolized by γ, the Lorentz factor is mathematically represented by

where v = velocity of the particle and c = speed of light. Relativistic momentum, p, can therefore be expressed as the product of velocity and relativistic mass : p = γmv. A non-circular and sound-enough derivation of relativistic mass, γm, is found here. As for the term γ itself, although it was originally conceived as a “fudging factor” to help experimental results agree with the flawed ether-in-space theory, it happens to be consistent with the fact that the speed of light does not vary, regardless of its source’s speed.

Imagine a traveller aboard a moving train,throwing a ball upwards to the ceiling and measuring the time it takes to return to the traveller. Now imagine someone outside the train standing still, call him Stillo. Stillo also measures the time for the traveller to get the ball back. But he observes a longer journey for the ball because the traveller will have moved horizontally across him while the ball is in flight. But because the train’s velocity adds to the horizontal velocity of the ball, the longer journey divided by the higher velocity yields the same time the traveller measured. But if you replace the ball with a beam from a flashlight, from both points of view, the velocity of light unlike that of the ball will remain the same. So the time measured by Stillo will have to be longer. The simple combination of the Pythagorean theorem and high school algebra will yield the above factor for the longer time observed.

from Serway’s Physics Text (see sources)

The traveller measures a total time of Δt’ = 2d/c, so d = cΔt’ /2. From the point of view of Stillo:

(cΔt/2)² = (vΔt/2)² + (d)²,                          where Δt =  time elapsed for Stillo

Substituting for d to introduce the traveller’s expression for time and eliminating the common denominator of 4, we get

c²Δt² =  v²Δt² + c²Δt’²

Transposing, factoring and isolating Δt², we get,

Δt² =c²Δt’²/(c² -v²)

Square rooting both sides and simplifying we obtain,

Δt  = Δt’ /√(1 -v²/c²)

or Δt  = γΔt’

Now we understand the roots, no pun intended, of γ, we can embark upon a little journey that will help us represent the total relativistic energy of an electron before and after it collides with x-rays. It will also provide us with a way of representing the momentum of a photon, which we mentioned has no mass. The formulas yielded will help us predict how the wavelength of the x-ray photon changes simply from measuring the angle of the scattered  x-ray. And with the mere knowledge of the original wavelength and the angle, with we could also use a similar analysis to predict the angle at which the electron is scattered.

That journey begins by differentiating relativistic momentum with respect to velocity:

(d/dv)[mv/√(1 -v²/c²)]

After applying the division rule and simplifying we will obtain

dp/dv = m(1 -v²/c²)^-3/2

The work done to bring an object from rest to a certain velocity is essentially equal to the kinetic energy the object will attain.  Work is the product of force and displacement, while force is the rate of change of momentum with respect to time.

But the problem is we can’t integrate an expression that has dt and dx . The chain rule gets us around the problem:

∫ (dp/dt)dx = ∫(dp/dv)(dx/dt)dv

Since the rate of change of displacement with restpect to time is velocity, the integral for kinetic energy will become

∫ (dp/dv) v dv . Substituting what we obtained for dp/dv

K.E  = ∫ m(1 -v²/c²)^-3/2 v dv. Letting u = 1 -v²/c², then du = -2v/c²dv  and integrating from 0 to v we obtain

K.E  = mc²/√(1 -v²/c²)  – mc² = γmc² – mc²

Rearranging,

γmc² = K.E + mc²

The equation reveals that the total relativistic energy is the sum of its kinetic energy and rest-mass energy.

The similar formulas E_total = γmc² and p =γmv allow us to temporarily eliminate m and γ to obtain an expression for velocity:

v = pc²/E

Returning to the expression for γ, 

If we square γ and multiply it by the new denominator, c² – v², we will get c².

So γ²(c² – v²) = c².

From the total energy formula we notice that γ =E_total/ mc² or γ² =(E_total)²/ (mc²)²

Substituting v = pc²/E and the latest expression for γ² into γ²(c² – v²) = c², we get

(E_total)² = (mc²)² + p²c²                                                             …equation(1)

A particle at rest has no momentum, so the above equation simplifies to

E = mc²   ,                                                                                  …equation (2)

which is a confirmation of what rest energy is equivalent to. But for a massless photon the above expression becomes E = pc.                        …equation(3)

In the photoelectric effect, electrons are emitted from a material when light shines on it. Such experiments in the late 19th century revealed that the intensity of light did not influence whether an electron was jarred out or not. If too long a wavelength was used, it was a lost cause, regardless of how bright the light was. Instead the correct wavelength (λ) would provide the necessary momentum to knock an electron loose. Since p = h/λ and c/λ = f, where f = frequency, the formula E = pc is consistent with Planck’s equation E = hf. The energy of the bundles that jar electrons loose are quantized. If those bundles or photons do not have sufficient energy (by having the wrong frequency) their collisions will be fruitless.

More convincing evidence for the existence of photons came in 1923.  Compton scattering is similar to the photoelectric effect, but it usually involves shorter wavelengths of incident radiation. With more energy the radiation is not entirely transferred to the ejected electron; most is scattered at an angle. According to classical theory the electron released from an atom of the material would move in the same direction as the original electromagnetic wave and the latter’s final wavelength would depend on the exposure time and on the original wavelength. But if photons existed, then the wavelength of the scattered photon would only depend on the scattered angle and the electron would also be scattered at an angle as shown in the diagram below.

from hyperphysics.phy-astr.gsu.edu

Since the electron could reach a relativistic speed, Compton derived an expression consistent with relativistic energy and with the photon theory. If the expression correctly predicted the photon’s final wavelength from experiment, then it would validate the existence of photons.

To derive the expression, Compton first used the conservation of momentum. From the diagram we see that the original vector for the momentum of the photon will equal the sum of the vectors of the recoiling electron and of the scattered photon. The scalar equivalent of that expression can be obtained by isolating the vector of the electron’s momentum and then squaring both sides to obtain:

p_e² =  p_i²-2p_ip_ecosθ + p_f²                                                      …equation(4)

where p_e = recoiled electron’s momentum; p_i = initial photon’s momentum; p_f = photon’s final momentum

Next he applied the conservation of energy. The energy of the original photon (equation 3) plus the rest mass (equation 2) of the electron (originally not moving out of the material) would be equal to the sum of the scattered photon’s energy and the total energy (obtained from equation 1) of the recoiled electron .

p_ic + m_ec² = p_fc + √[(m_ec²)² + p_e²c² ]                                  …equation(5)

If you square both sides of equation(5) and solve for p_e²  you get

p_e²= p_i² + 2m_ep_ic  – 2p_ip_f – 2m_ep_fc + p_f² .                           …equation(6)

Next we can equate equation (4) to equation (6), and after cancelling common terms and factoring we obtain,

m_ec(p_i-p_f) = p_ip_f(1 – cosθ)

since p = h/λ, we could make the substitutions for the initial and final momenta, multiply through by  λ_iλ_f , and upon isolating the (λ_f – λ_i )term we obtain

λ_f – λ_i = Δλ = (h/m_ec)(1 – cosθ)                                               …equation(7)

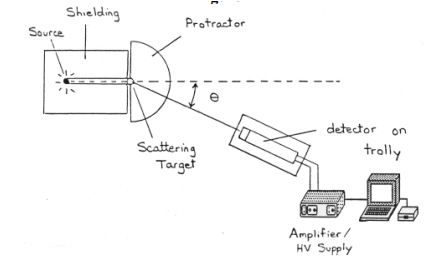
A common undergraduate lab involves a modified version of the Compton experiment. It uses gamma rays from a radioactive isotope. Lead is used to shield the source in order to minimize students’ exposure to radiation. They vary the angle and the detector of scattered photons is connected to a computer. From analyzing the peaks of the graph, the students get the energy from which they can obtain the wavelength. The data reveals that the change in wavelength is indeed dependent only on the scattering photon’s angle. It confirms that in this type of experiment the x-rays act as photons and not as electromagnetic waves.

The data can also be used to work backwards to obtain the mass of the electron. As for the scattering angle, φ ,of the electron, the only formula I was able to find was in Wikipedia:   cotφ = (1+hf /(m_ec²)) tan(θ/2). Since they do not reveal its derivation , I’ll indulge you by suggesting you write separate conservation of momenta expressions for the x and y components of the momentum of the electron. If you isolate cosφ and sinφ in the x and y expressions respectively and divide them, the p_e term cancels and you get:

cotφ = λ_f/(λ_isinθ) – cotθ                                                            …equation(8)

If you want to eliminate λ_f since f in the Wiki formula only has f, the photon’s initial frequency, you can simply solve for λ_f in equation (7) and then substitute it into equation(8) to obtain 

cotφ = (h/(m_ec)+λ_i )(1 – cosθ) /(λ_isinθ)                                       …equation(9)

There’s an identity in trigonometry where (1 – cosθ) /sinθ = tan(θ/2). Substituting into equation (9), we get

cotφ = (h/(m_ec)+λ_i )tan(θ/2)/ λ_i 

If you finally divide each of the bracketed terms by the numerator and replace 1/λ_i with its equivalent, f/c, we obtain Wiki’s formula:

cotφ = (1+hf /(m_ec²)) tan(θ/2)

If, after all those mathematical detours, you are still with me, I want to end by pointing out that Compton scattering is still an area of active research. After all, it is the most dominant interaction when photons of 0.2 MeV (hard X-rays) to 10 MeV (gamma rays) collide with elements with atomic numbers below 30 such as the ones found in human tissue. Many other investigations involving Compton scattering are being done at the nucleon level.

 

Sources:
Radiation Risk From Medical Imaging

https://www.ncbi.nlm.nih.gov/pmc/articles/PMC2996147/#R3

Compton scattering hyperphysics.phy-astr.gsu.edu

Compton scattering en.wikipedia.org/wiki/Compton_scattering

Physics for Scientists and Engineers With Modern Physics. Serway. Saunders 3rd Edition. 1992

Principles of Radiation Interactions https://ocw.mit.edu/courses/nuclear-engineering/22-55j-principles-of-radiation-interactions-fall-2004/lecture-notes/ener_depo_photon.pdf